Pinching4 Material: Difference between revisions

From OpenSeesWiki
Jump to navigation Jump to search
No edit summary
No edit summary
 
(3 intermediate revisions by the same user not shown)
Line 78: Line 78:
EXAMPLE:
EXAMPLE:


[[Pinching4MaterialExample]] - the original file (has some errors)
[[Pinching4MaterialExample]]  
[[Pinching4MaterialExample_Corrected]]  


----
----
Line 93: Line 92:
REFERENCES:
REFERENCES:


[http://peer.berkeley.edu/publications/peer_reports/reports_2003/0310.pdf PEER 2003/10]




Latest revision as of 14:12, 7 April 2012



This command is used to construct a uniaxial material that represents a 'pinched' load-deformation response and exhibits degradation under cyclic loading. Cyclic degradation of strength and stiffness occurs in three ways: unloading stiffness degradation, reloading stiffness degradation, strength degradation.

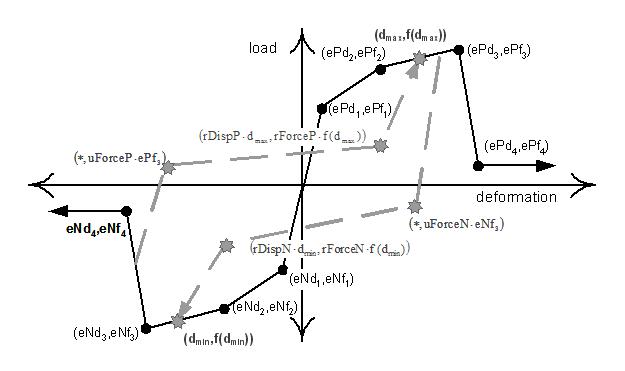
uniaxialMaterial Pinching4 $matTag $ePf1 $ePd1 $ePf2 $ePd2 $ePf3 $ePd3 $ePf4 $ePd4 <$eNf1 $eNd1 $eNf2 $eNd2 $eNf3 $eNd3 $eNf4 $eNd4> $rDispP $rForceP $uForceP <$rDispN $rForceN $uForceN > $gK1 $gK2 $gK3 $gK4 $gKLim $gD1 $gD2 $gD3 $gD4 $gDLim $gF1 $gF2 $gF3 $gF4 $gFLim $gE $dmgType
$matTag integer tag identifying material
$ePf1 $ePf2 $ePf3 $ePf4 floating point values defining force points on the positive response envelope
$ePd1 $ePd2 $ePd3 $ePd4 floating point values defining deformation points on the positive response envelope
$eNf1 $eNf2 $eNf3 $eNf4 floating point values defining force points on the negative response envelope
$eNd1 $eNd2 $eNd3 $eNd4 floating point values defining deformation points on the negative response envelope
$rDispP floating point value defining the ratio of the deformation at which reloading occurs to the maximum historic deformation demand
$fFoceP floating point value defining the ratio of the force at which reloading begins to force corresponding to the maximum historic deformation demand
$uForceP floating point value defining the ratio of strength developed upon unloading from negative load to the maximum strength developed under monotonic loading
$rDispN floating point value defining the ratio of the deformation at which reloading occurs to the minimum historic deformation demand
$fFoceN floating point value defining the ratio of the force at which reloading begins to force corresponding to the minimum historic deformation demand
$uForceN floating point value defining the ratio of strength developed upon unloading from negative load to the minimum strength developed under monotonic loading
$gK1 $gK2 $gK3 $gK4 $gKLim floating point values controlling cyclic degradation model for unloading stiffness degradation
$gD1 $gD2 $gD3 $gD4 $gDLim floating point values controlling cyclic degradation model for reloading stiffness degradation
$gF1 $gF2 $gF3 $gF4 $gFLim floating point values controlling cyclic degradation model for strength degradation
$gE floating point value used to define maximum energy dissipation under cyclic loading. Total energy dissipation capacity is defined as this factor multiplied by the energy dissipated under monotonic loading.
$dmgType string to indicate type of damage (option: "cycle", "energy")


NOTES:

Damage Models:

Stiffness and strength are assumed to deteriorate due to the imposed "load" history. The same basic equations are used to describe deterioration in strength, unloading stiffness and reloading stiffness:

<math>k_i = k_0(1 -\delta k_i)</math>

where <math>k_i</math> is the unloading stiffness at time <math>t_i</math>, <math>k_0</math> is the initial unloading stiffness (for the case of no damage), and <math>\delta k_i</math>(defined below) is the value of the stiffness damage index at time <math>t_i</math>.

<math>d_{\text{max i}} = d_{\text{max 0}}(1 -\delta d_i)</math>

where <math>d_{\text{max i}}</math> is the deformation demand that defines the end of the reload cycle for increasing deformation demand, <math>d_{\text{max 0}} </math> is the maximum historic deformation demand (which would be the deformation demand defining the end of the reload cycle if degradation of reloading stiffness is ignored), and <math>\delta d_i</math> (defined below) is the value of reloading stiffness damage index at time <math>t_i</math>.

<math>f_{\text{max i}} = f_{\text{max 0}}(1 -\delta f_i)</math>

where <math>f_{\text{max i}}</math> is the current envelope maximum strength at time <math>t_i</math>, <math>f_{\text{max 0}} </math> is the initial envelope maximum strength for the case of no damage, and <math>\delta f_i</math> (defined below) is the value of strength value index at time <math>t_i</math>.

The damage indices <math>\delta k_i</math>, <math>\delta d_i</math>, and <math>\delta f_i</math>, may be defined to be a function of displacement history only ($dmgType = "cycle") or displacement history and energy accumulation ($dmgType = "energy"). For either case, all of the damage indices are computed using the same basic equation.

If the damage indices are assumed to be a function of displacement history and energy accumulation, the unloading stiffness damage index, <math>\delta k_i</math> is computed as follows:


<math>\delta k_i = \left( \text{gK1} (d_{max})^\text{gK3} + \text{gK2} \left (\frac{E_i}{E_\text{monotonic}} \right )^\text{gK3} \right ) <= \text{gKLim}</math>

where

<math>(d_{max} = \text{max} \left[ \frac{d_\text{max i}}{\text{def}_\text{max}}, \frac{d_\text{min i}}{\text{def}_\text{min}} \right ]</math>

EXAMPLE:

Pinching4MaterialExample


DESCRIPTION:

Stiffness and strength are assumed to deteriorate due to the imposed "load" history. The same basic equations are used to describe deterioration in strength, unloading stiffness and reloading stiffness:


REFERENCES:

PEER 2003/10



Code Developed by: Nilinjan Mitra, University of Washington

Retrieved from "https://opensees.ist.berkeley.edu/wiki/index.php?title=Pinching4_Material&oldid=9436"